collectionsbooster.blogg.se - Run a t-test in excel for mac

RUN A T TEST IN EXCEL FOR MAC DOWNLOAD

Step 4: Select Analysis ToolPak and click OK. Step 3: Select Excel Add-ins and click Go If you do not find this option in your excel, follow the below steps to unhide this. We can run the T.TEST using the analysis tool pack located under the Data ribbon tab. I want to find if the mean of two department’s salaries is significantly different are not.Īpply the T.TEST function to see the difference. I have the salary numbers of two different departments. Otherwise, the two means are not significantly different from each other. If the P-value is <0.05, we can come to the conclusion that the two sets of data have a different mean. The returned value is generally called as the P-value. The third test is a type of Two Sample unequal variance. The second test is a type of Two Samples equal variance.

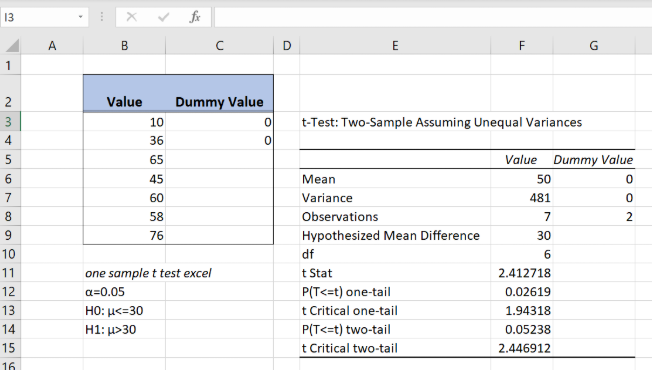
I need to run T.TEST to find is there a significant difference between these two groups. I have Group 1 and Group 2 test scores of a classroom. The functionality of the T.TEST can be best explained by using an example dataset to get the logic of the T.TEST.

RUN A T TEST IN EXCEL FOR MAC DOWNLOAD

Since this interval includes zero, and because $p \gt 0.05$, we do not reject the null hypothesis.You can download this T.TEST Function Excel Template here – T.TEST Function Excel Template Example #1 The 95% confidence interval around the estimated mean difference is. Evaluating this against a $t$-distribution with 99 degrees of freedom, we get a (two-sided) $p$-value of 0.123, not enough to be statistically significant.

We see that the difference in means is 3.52, which results in a $t$-statistic equal to 1.56. (2-tailed) gives the $p$-value, which is 0.123 $df$ gives the degrees of freedom, which is 99.The $t$ column gives the $t$-statistic, which is 1.557.The 95% Confidence Interval of the Difference is.IQ at time 1 consists of a sample with size equal to 100, mean of 103.2734, standard deviation of 17.10415, and a standard error mean of 1.71041 ( $s/ \sqrt$, which is 2.62317 The first is summary statistics for each variable. Because this includes zero (equivalent to $p 0.05 $), we do not reject the null. We also see that the 95% confidence interval around the mean difference is. This is greater than 0.05, so we fail to reject the null-hypothesis of no difference. When evaluated against a $t$-distribution with 198 degrees of freedom, we get a $p$-value of 0.204. If we use unequal variances, the results are treated the same, but a slightly different formula is used to calculate the values. The 95% Confidence Interval of the Difference is, or with unequal variances.Error Difference is 2.23042 (2.21101 unequal variances) Mean Difference gives the difference between the two sample means, which is -2.84.

(2-tailed) gives the $p$-value which is 0.204 (0.200 unequal variances)

$df$ gives the degrees of freedom which is 198 (194.268 unequal variances).

The $t$ column gives the $t$-statistic which is -1.275 (-1.286 unequal variances).

When this is the case, there is a version of the $t$-test that adjusts for the unequal variance, which is also displayed. The null hypothesis is that the assumption is met, so a significant result ( $p<0.05$) means that the assumption is violated. The Levene’s test for equality of variances allows us to check the assumption. Note that an assumption for the independent samples $t$-test is that the two groups have equal variance, but that assumption is often violated. The second table is the result of the $t$-test. For females, we had a sample size of $n = 104$ with a mean IQ of 106.4, a standard deviation of 17.31, and a standard error mean of 1.7. For males, we had a sample of $n = 95$ with a mean IQ of 103.56, a standard deviation of 13.88 and a standard error mean of 1.42. The first is summary statistics for each sample.